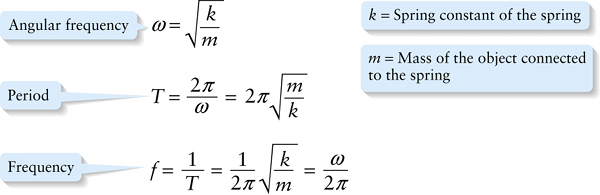Chapter 12. Angular frequency, period, and frequency for a block attached to an ideal spring (12-11)
Question
74ZQsRWNs5eaxFMtu1ST1zsHSXQD2A/8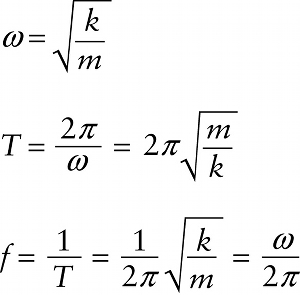
Question
i3GYZUGh83sgFQrZ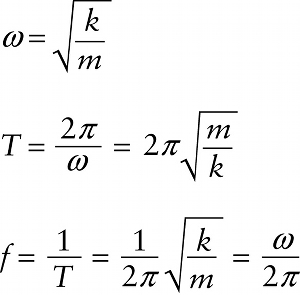
Question
6AjRK1rC+/08Tcq8CY6Akw==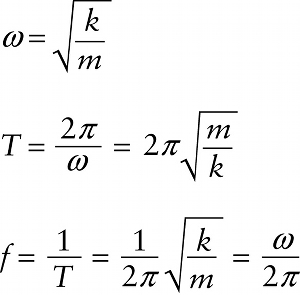
Review
We’ve seen that the period T of the oscillation in Figure 12-4b is the same as the time \(T\) that it takes the object in uniform circular motion in Figure 12-4a to travel once around the circle. The angular speed \(\omega\) equals \(2\pi/T\), so \(T\) equals \(2\pi/\omega\). Using Equation 12-10 for the value of \(\omega\) along with Equation 12-1 for the relationship between period \(T\) and frequency \(f\), we get the following results for the oscillations of a block of mass \(m\) attached to an ideal, Hooke’s law spring of spring constant \(k\):