Chapter 14. Root-mean-square speed of molecules in an ideal gas (14-15)
Question
RObeOV8tQNcveiWfYXuxCr+tCAp6X8z6yknnPuf3dSAWtF6gVZGyMPJWsfJYLRHNXkWqDBkWL8b0rYNPryBu+DqnPTvKh+n2
Question
/9IODz7PTiIPwtNrsDRjeLOlsZnOKcIzkZAAnM9HT0bYowYc
Question
A1DNMDK9RQNrFNSE8Xl81QraJRihc3su/kb9bS2+9b6RpFW0So/hlM5DXrZfc4EgDeKiP+U8vfkQr+Pi
Question
DhJyyMOVyE6vl/ynKzCF9Y1Iot04f/zGep9N+KasodSyYfYpQn8lJd8A++vtdJ0r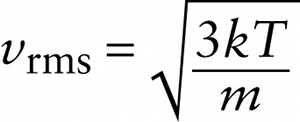
Review
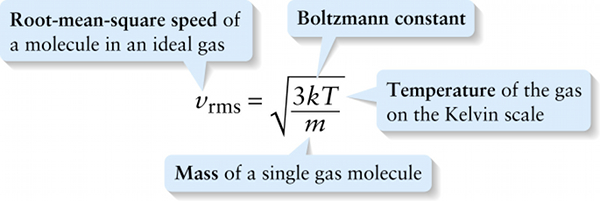
A measure of how fast gas molecules move on average is the \(\textbf{root-mean-square speed}\) or \(\textbf{rms speed}\). To see how this is defined, first imagine finding the average value of \(v^2\), the square of the speed \(v\), for all the molecules in a gas that have a given mass \(m\). This is the quantity that we’ve called \((v^2)_{\mathrm{average}}\). Another word for average is mean, which is why \((v^2)_{\mathrm{average}}\) is called the mean-square of the speed. The root-mean-square speed is the square root of the mean-square:
\(v_{\mathrm{rms}} = \sqrt{(v^2)_{\mathrm{average}}}\)
Note that \((v^2)_{\mathrm{average}}\) has units of m/s. From Equation 14-13, \((1/2)m(v^2)_{\mathrm{average}} = (3/2)kT\), so \((v^2)_{\mathrm{average}} = 3kT/m\). If we substitute this into Equation 14-14 we get: